« Previous « Start » Next »
12 Constrained Global Optimization
In
glc_prob there are 30 global mixed-integer nonlinear programming
test problems with sizes to 20 variables and 5 constrains.
In order to define the problem
n and solve it execute the following in Matlab:
Prob = probInit('glc_prob',n);
Result = tomRun('',Prob);
The basic structure of a constrained global optimization problems is the following
|
|
|
f(x) |
| |
|
| s/t |
| −∞ < |
xL |
≤ |
x |
≤ |
xU |
< ∞ |
| |
bL |
≤ |
A x |
≤ |
bU |
|
| |
cL |
≤ |
c(x) |
≤ |
cU, |
xj 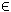 N N  j j 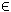 I, I, |
|
|
(14) |
where
x,
xL,
xU 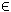 Rn
Rn,
f(
x)
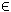 R
R,
A
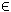 Rm1 × n
Rm1 × n,
bL,
bU 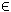 Rm1
Rm1
and
cL,
c(
x),
cU 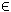 Rm2
Rm2. The variables
x 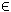 I
I,
the index subset of 1,...,
n, are restricted to be integers.
The following files are required to define a problem of this category in TOMLAB.
File: tomlab/quickguide/glcQG_f.m, glcQG_c.m
f: Function
c: Constraints
An example of a problem of this class,
(that is also found in the TOMLAB quickguide) is glcQG:
File: tomlab/quickguide/glcQG.m
% glcQG is a small example problem for defining and solving
% constrained global programming problems using the TOMLAB format.
Name = 'Hock-Schittkowski 59';
u = [75.196 3.8112 0.0020567 1.0345E-5 6.8306 0.030234 1.28134E-3 ...
2.266E-7 0.25645 0.0034604 1.3514E-5 28.106 5.2375E-6 6.3E-8 ...
7E-10 3.405E-4 1.6638E-6 2.8673 3.5256E-5];
x_L = [0 0]'; % Lower bounds for x.
x_U = [75 65]'; % Upper bounds for x.
b_L = []; b_U = []; A = []; % Linear constraints
c_L = [0 0 0]; % Lower bounds for nonlinear constraints.
c_U = []; % Upper bounds for nonlinear constraints.
x_opt = [13.55010424 51.66018129]; % Optimum vector
f_opt = -7.804226324; % Optimum
x_min = x_L; % For plotting
x_max = x_U; % For plotting
x_0 = [90 10]'; % If running local solver
Prob = glcAssign('glcQG_f', x_L, x_U, Name, A, b_L, b_U, ...
'glcQG_c', c_L, c_U, x_0, ...
[], [], [], [], ...
[], x_min, x_max, f_opt, x_opt);
Prob.user.u = u;
Prob.optParam.MaxFunc = 1500;
Result = tomRun('glcFast', Prob, 1);
%Result = tomRun('glcSolve', Prob, 1);
%Result = tomRun('lgo', Prob, 1);
%Result = tomRun('oqnlp', Prob, 1);
« Previous « Start » Next »
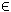 Rn, f(x)
Rn, f(x) 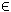 R, A
R, A
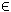 Rm1 × n, bL,bU
Rm1 × n, bL,bU 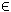 Rm1
and cL,c(x),cU
Rm1
and cL,c(x),cU 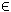 Rm2. The variables x
Rm2. The variables x 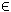 I,
the index subset of 1,...,n, are restricted to be integers.
I,
the index subset of 1,...,n, are restricted to be integers.